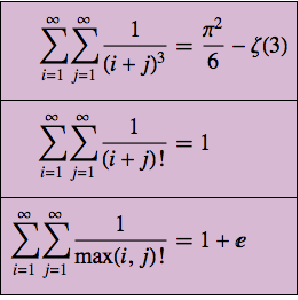Use Feynman's Trick for Evaluating Integrals 

Inactive can be used to derive identities by applying standard techniques such as Feynman's trick of differentiating under the integral sign.

Derive a closed form for 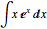by analyzing .

In[1]:=

X int = Inactivate[D[Integrate[E^(a x), x], a], D | Integrate]

Out[1]=

First differentiating with respect to 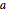at produces the desired integral.

In[2]:=

X lhs = Activate[int, D] /. a -> 1

Out[2]=

If the integration is done first, the integral is simple.

In[3]:=

X Activate[int, Integrate]

Out[3]=

The derivative with respect to at is also straightforward.

In[4]:=

X Activate[int, Integrate]; Activate[%, D]

Out[4]=

In[5]:=

X int = Inactivate[D[Integrate[E^(a x), x], a], D | Integrate]; Activate[%, D]; rhs = % /. a -> 1 // FullSimplify

Out[5]=

Equating the two expressions produces the answer.

In[6]:=

X lhs == rhs // TraditionalForm

Out[6]//TraditionalForm=

Verify the answer.

In[7]:=

X lhs == rhs // TraditionalForm; Activate[%] // Simplify

Out[7]=