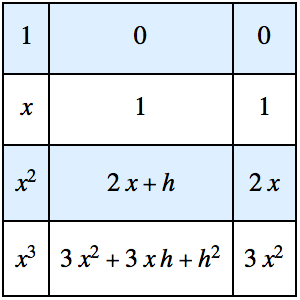
Find the Charge Distribution on a Sphere
Find the positions that minimize the Coulomb potential for equally charged particles free to move on a sphere. This is the equilibrium charge distribution.
Denote by n the number of particles.
In[1]:=
n = 50;Let 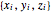 be the Cartesian coordinates of the
be the Cartesian coordinates of the 
![]() particle.
particle.
In[2]:=
vars = Join[Array[x, n], Array[y, n], Array[z, n]];The goal is to minimize the Coulomb potential.
In[3]:=
potential =
Sum[((x[i] - x[j])^2 + (y[i] - y[j])^2 + (z[i] - z[j])^2)^-(1/
2), {i, 1, n - 1}, {j, i + 1, n}];Since the particles are on a sphere, their coordinates must satisfy unit-magnitude constraints.
In[4]:=
sphereconstr = Table[x[i]^2 + y[i]^2 + z[i]^2 == 1, {i, 1, n}];Choose initial points on the sphere at random using spherical coordinates.
In[5]:=

rpts = ConstantArray[1, n];
thetapts = RandomReal[{0, Pi}, n];
phipts = RandomReal[{-Pi, Pi}, n];
spherpts = Transpose[{rpts, thetapts, phipts}];Transform the initial points to Cartesian coordinates.
In[6]:=
cartpts = CoordinateTransform["Spherical" -> "Cartesian", spherpts];Rearrange the initial points to match the variables' ordering.
In[7]:=
initpts = Flatten[Transpose[cartpts]];Minimize the Coulomb potential subject to the sphere constraint.
In[8]:=
sol = FindMinimum[{potential, sphereconstr}, Thread[{vars, initpts}]];Extract from the solution the equilibrium positions of the particles.
In[9]:=
solpts = Table[{x[i], y[i], z[i]}, {i, 1, n}] /. sol[[2]];Plot the result.
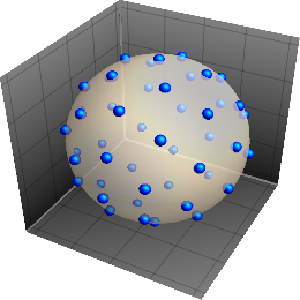
In[10]:=

Show[ListPointPlot3D[solpts,
PlotRange -> {{-1.1, 1.1}, {-1.1, 1.1}, {-1.1, 1.1}},
PlotStyle -> {{PointSize[.03], Blue}}, AspectRatio -> 1,
BoxRatios -> 1, PlotLabel -> "Particle Distribution"],
Graphics3D[{Opacity[.5], Sphere[]}]]Out[10]=